The Speed of Sound Finally Bounded by Fundamental Constants

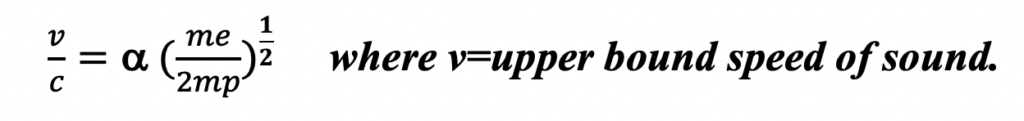
The scientists went on to test the validity of their equation on a variety of 36 elemental solid mediums, where they plotted a log-log plot of the speed of sound in that solid as a function of its atomic mass. Empirically, they ascertained that the speed of sound decreases when atomic mass increases, meaning that the upper bound of the speed of sound is to be found in solid hydrogen with strong metallic bonding (since it has the smallest atomic mass). Metallic hydrogen only exists at megabar pressures and is dynamically volatile at ambient pressure where molecular formation occurs. Thus, the team encountered an impediment; they could not source actual atomic hydrogen to test their hypothesis. Notwithstanding, they found a solution (admittedly imperfect) by conducting first-principles computations for I41/amd structure, the closest proxy they could find for atomic hydrogen, and performed the computations for this new structure in the pressure range of 250 to 1000GPa. They then graphed the speed of sound of this structure as a function of both pressure and density. These results agreed with the earlier prediction that sound should propagate at speeds up to 36,000 m/s. (Trancheko et al., 2020).

The implications of these results have already been perceived in the field of physics; for instance, this “new” upper bound of the speed of sound, which is twice the speed at which sound travels through very stiff diamond, has reconstructed how we view sound propagation. It sheds light on the usefulness of fundamental constants in defining the real-world behavior of matter and energy. In light of this, Vadim Brazhkin-one of the team of scientists in this experiment had already published a paper which revealed that the universal lower limit on viscosity is again denoted in terms of the proton-to-electron mass ratio and Planck’s constant. (Trachenko & Brazhkin, 2020). The use of the fine structure constant and proton-to-electron mass ratio have far-reaching connections too, among them being that these constants may govern nuclear reactions that lead to the formulation of primary biochemical elements like Carbon. (Trancheko et al., 2020).

This analysis, in conjunction with their already established data on bounds for viscosity and diffusivity, sheds light on the importance of finding the bounds of condensed matter phases. To further cement this conclusion, Professor Kostya Trachenko, Professor of Physics at Queen Mary asserted, “We believe the findings of this study could have further scientific applications by helping us to find and understand limits of different properties such as viscosity and thermal conductivity relevant for high-temperature superconductivity, quark-gluon plasma and even black hole physics.” (Rainey, 2020)